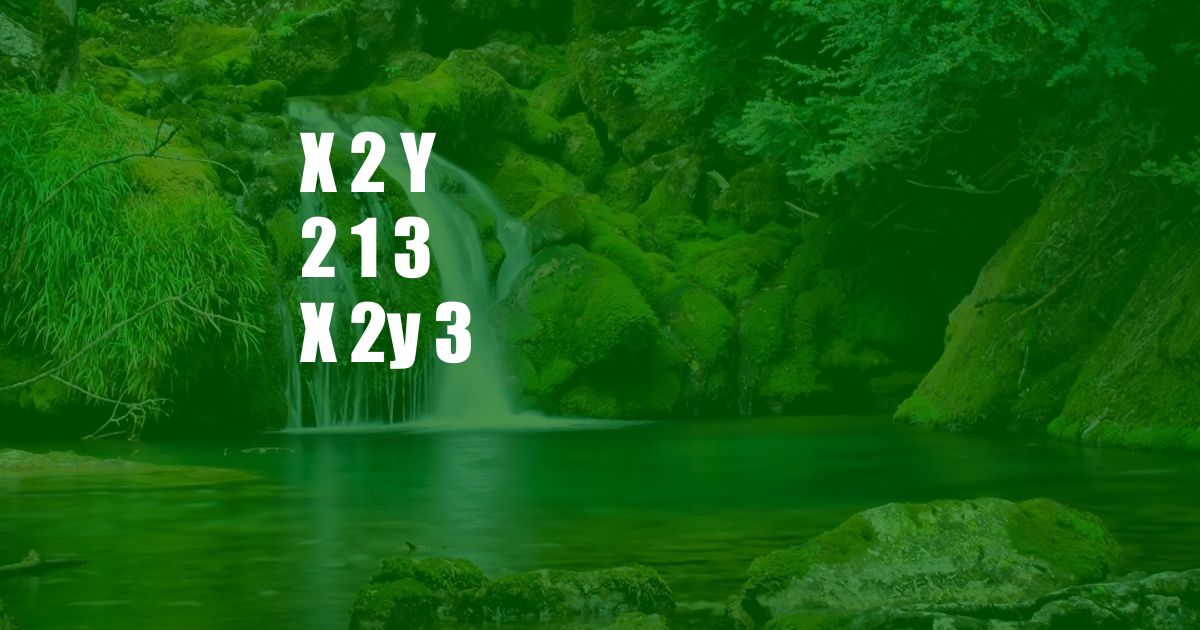
X 2 Y 2 1 3 X 2y 3

x^2 y^2 1 3 x^2y^3: Unraveling Algebraic Expressions

I’ve always had a love for mathematics. The precision, the logic, the beauty of it all. But I’ll admit, algebraic expressions like x^2 y^2 1 3 x^2y^3 used to intimidate me. They seemed like an impenetrable code, a language only the chosen few could comprehend. Until I finally decided to break them down, piece by piece, and discovered the elegant simplicity that lay beneath.

So if you’re struggling with algebraic expressions, know that you’re not alone. And if you’re ready to conquer them, I’m here to guide you through the journey. Let’s start by demystifying the terms.

Understanding the Building Blocks: Variables and Exponents

In an algebraic expression, variables represent unknown values, while exponents indicate how many times a variable should be multiplied by itself. In our case, we have the variables x and y, and the exponents 2 and 3.

So, x^2 means that x is multiplied by itself twice, which is the same as x * x. Similarly, y^3 means that y is multiplied by itself three times, or y * y * y. The constant term, 1, means just that – a value of 1. And finally, 3 x^2y^3 represents the product of 3 and x^2 y^3, which is written as 3 * x^2 * y^3.

Expanding and Simplifying Algebraic Expressions

Once we understand the building blocks, we can expand and simplify algebraic expressions. Expanding means multiplying out all the terms, while simplifying means combining like terms.

To expand x^2 y^2 1 3 x^2y^3, we multiply each term by every other term. This gives us the following result:

x^2 y^2 * 1 * 3 x^2y^3

= 3 x^4 y^5

And that’s it! We have simplified the expression down to a single term.

Exploring Real-World Applications

Algebraic expressions are more than just abstract concepts; they have real-world applications in fields such as physics, engineering, and finance. For example, in physics, the equation F = ma describes the relationship between force (F), mass (m), and acceleration (a). In finance, the formula A = P(1 + r/n)^(nt) calculates the future value (A) of an investment (P) over time (t), given an interest rate (r) and the number of compounding periods (n).

Tips for Mastering Algebraic Expressions

here’s a few tips to help you become more comfortable with algebraic expressions:

• Practice regularly: The more you work with algebraic expressions, the more comfortable you’ll become.
• Break down complex expressions: Don’t try to tackle everything at once. Start by breaking down the expression into smaller, more manageable chunks.
• Use a calculator: Don’t be afraid to use a calculator to check your work or simplify complex calculations.

Frequently Asked Questions (FAQs)

1. What is an algebraic expression?

   An algebraic expression is a combination of variables, constants, and operations (such as addition, subtraction, multiplication, and division).

2. How do you simplify algebraic expressions?

   You can simplify algebraic expressions by combining like terms, expanding, and factoring.

3. What are some real-world applications of algebraic expressions?

   Algebraic expressions are used in many fields, including physics, engineering, and finance.

Conclusion

There you have it, a comprehensive guide to x^2 y^2 1 3 x^2y^3 and algebraic expressions in general. Whether you’re a student grappling with homework or a professional seeking to enhance your mathematical skills, I hope this article has provided you with the clarity and confidence to conquer these equations.

So, tell me, how do you feel about algebraic expressions now? Are you ready to embrace the challenge and uncover their hidden elegance? The journey to mathematical mastery starts here.